Here are some interesting objects made from paper. Something like this is a good project for a winter break. They're fun to work on, and satisfying to complete.

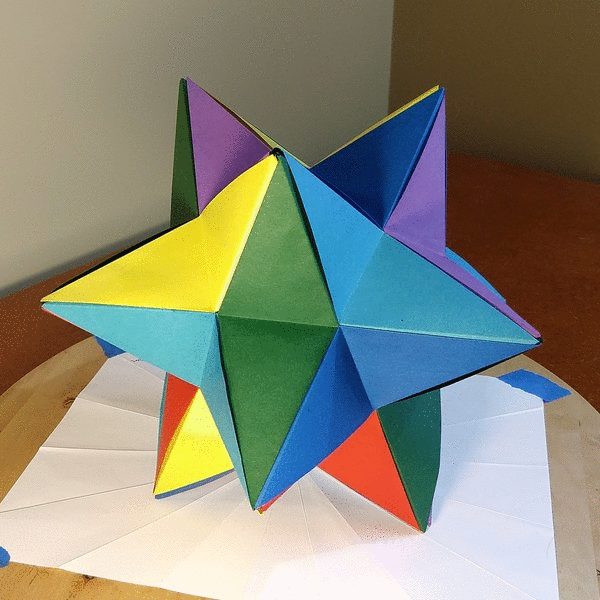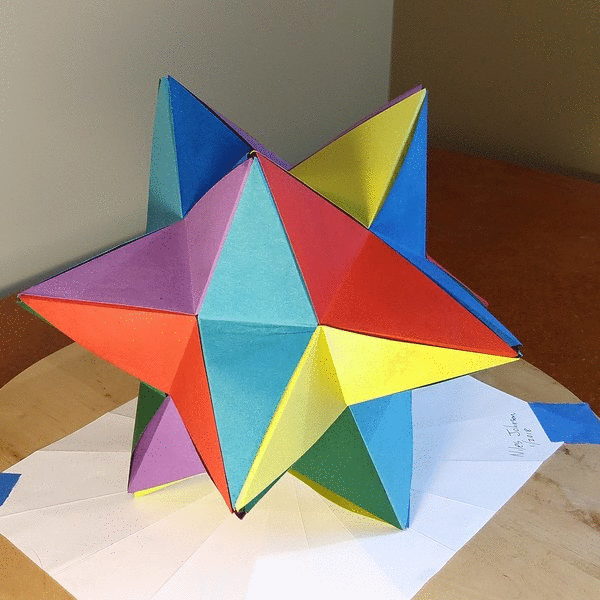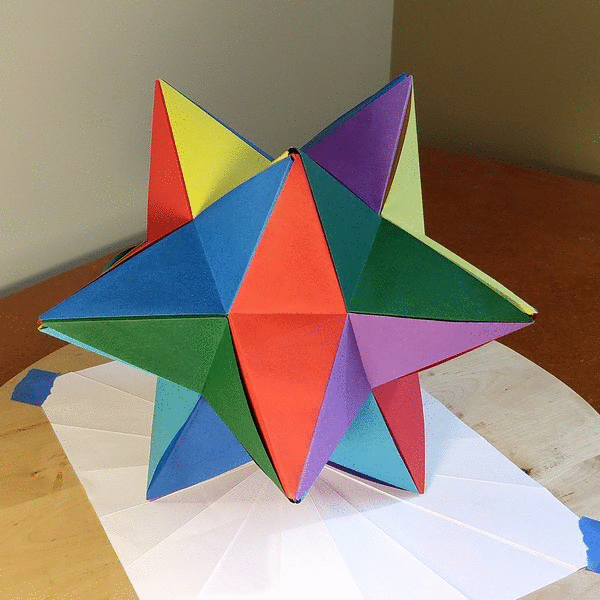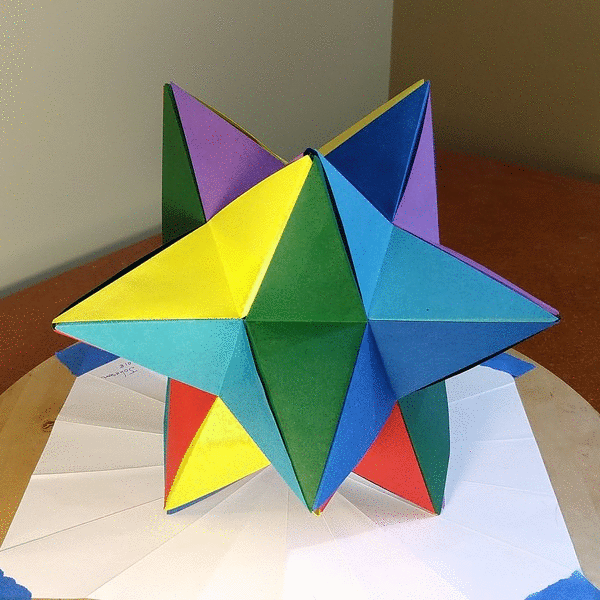
The Stellated Icosahedron

This is a stellated icosahedron made with 30 Sonobe modules in 6 colors. The colors are arranged in 6 interlocking bands, with 5 distinct colors meeting at each of 12 star-shaped faces. There are 20 triangular points in 10 antipodal pairs. These each have 3 colors, and the 10 pairs represent all possible choices of 3 colors.

You can find instructions online, but see my version for instructions about arranging the colors.

The Stellated Dodecahedron

This is a stellated dodecahedron made with 30 of M. Mukhopadhyay's super simple isosceles triangle module. It has the same coloring arangement as the stellated icosahedron above.

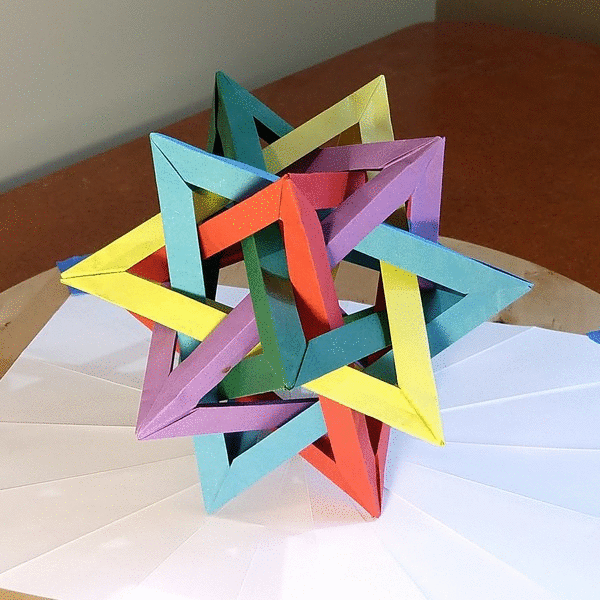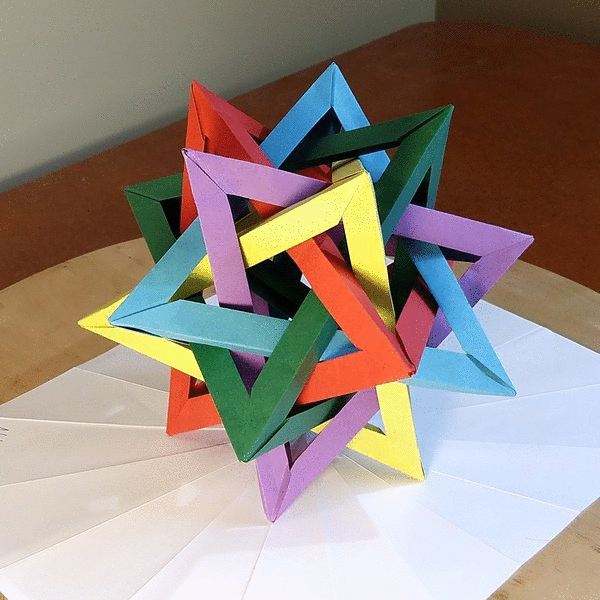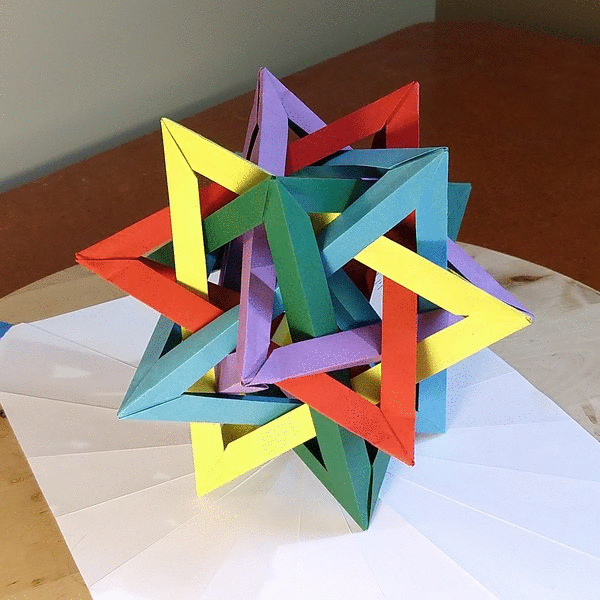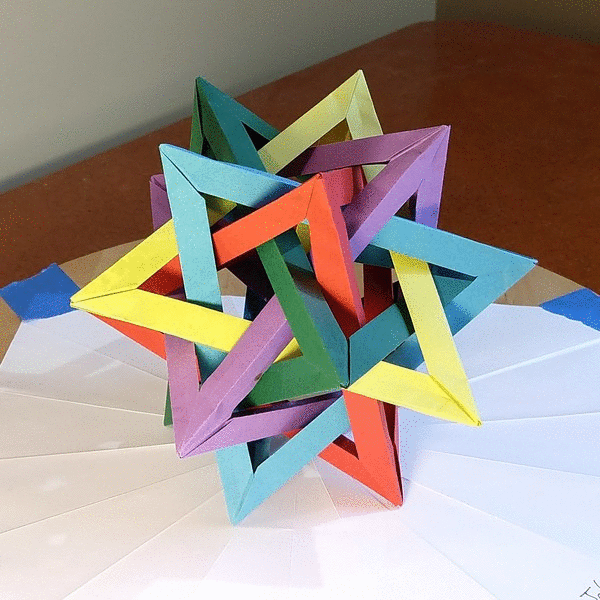
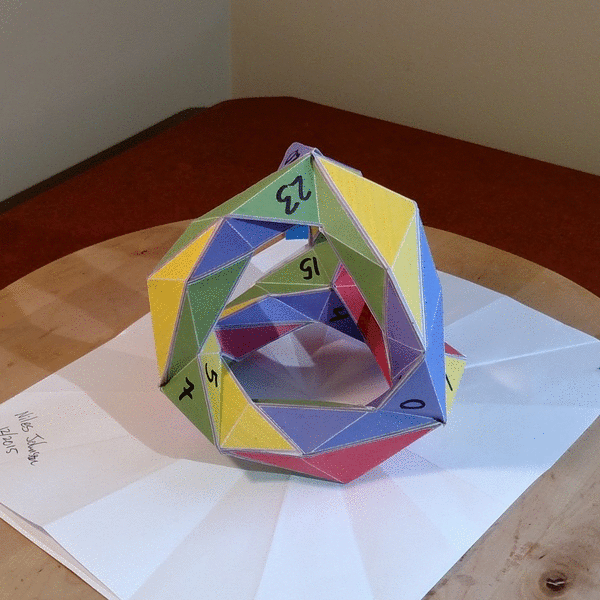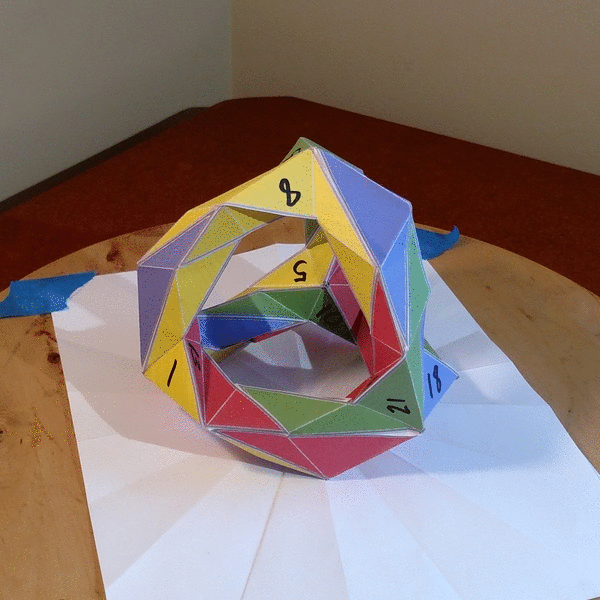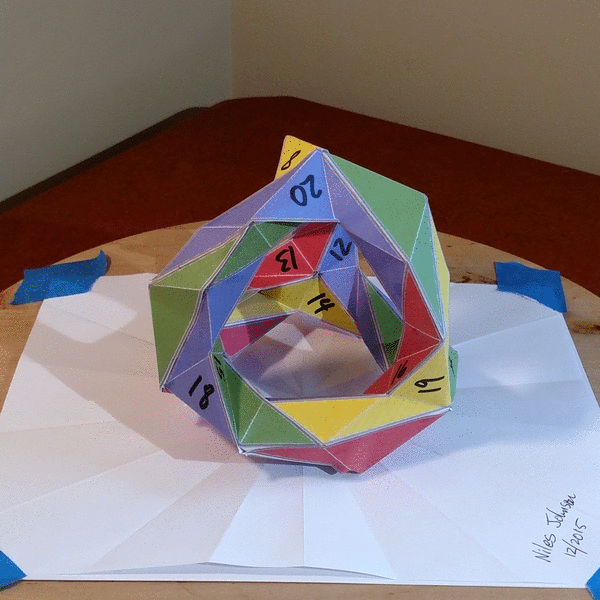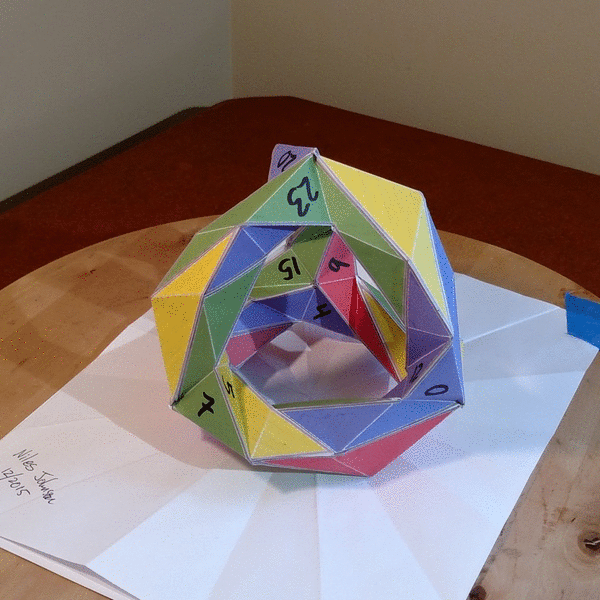
The Compound of Five Tetrahedra

This shows 5 interlocking tetrahedra arranged with their vertices forming a dodecahedron. Read more on the Wikipedia article. Click through for my construction notes.

The Klein Quartic

A Klein quartic made with 24 heptagons (7-sided shapes). In a 4-dimensional space (CP^2), this can be done with regular heptagons, and the shape has 168 symmetries. This is the largest possible for a 3-holed donught! To push the shape down to 3 dimensions destroys most of this symmetry, and distorts the regular heptagons severely. But by doing so we can construct it out of paper!

tags: papercraft | mathart