Research in Categorical Algebra
A bicategory is a kind of (weak) 2-category, and classical Morita theory can be phrased in terms of a classical example of a bicategory. This is the bicategory whose 0-cells are rings, 1-cells are bimodules, and 2-cells are bimodule homomorphisms. The unit 1-cell over a ring is that ring considered as a bimodule over itself, and composition of 1-cells is defined by the tensor product.
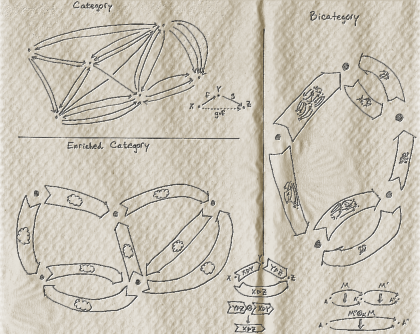
Napkin depiction of a category, an enriched category, and a bicategory.
A Morita equivalence of rings is simply an equivalence of 0-cells in this bicategory. That is, a 1-cell from one ring to another (a bimodule), with a 1-cell inverse, so that the composite (tensor product) of these two bimodules over one ring is isomorphic to the other, and vice-versa.
These projects develop 2-categorical algebra for applications in Brauer theory.
Modeling Stable One-Types
Joint with
Angélica Osorno
Theory and Applications
of Categories.
26 (2012) No. 20, pp 520--537.
arXiv 1201.2686:
[abs |
pdf]
Slides (JMM 2012, Boston)
Abstract:
Classification of homotopy $n$-types has focused on developing algebraic categories which are equivalent to categories of $n$-types. We expand this theory by providing algebraic models of homotopy-theoretic constructions for stable one-types. These include a model for the Postnikov one-truncation of the sphere spectrum, and for its action on the model of a stable one-type. We show that a bicategorical cokernel introduced by Vitale models the cofiber of a map between stable one-types, and apply this to develop an algebraic model for the Postnikov data of a stable one-type.Additional topics are currently work in progress; check back later for more information!
